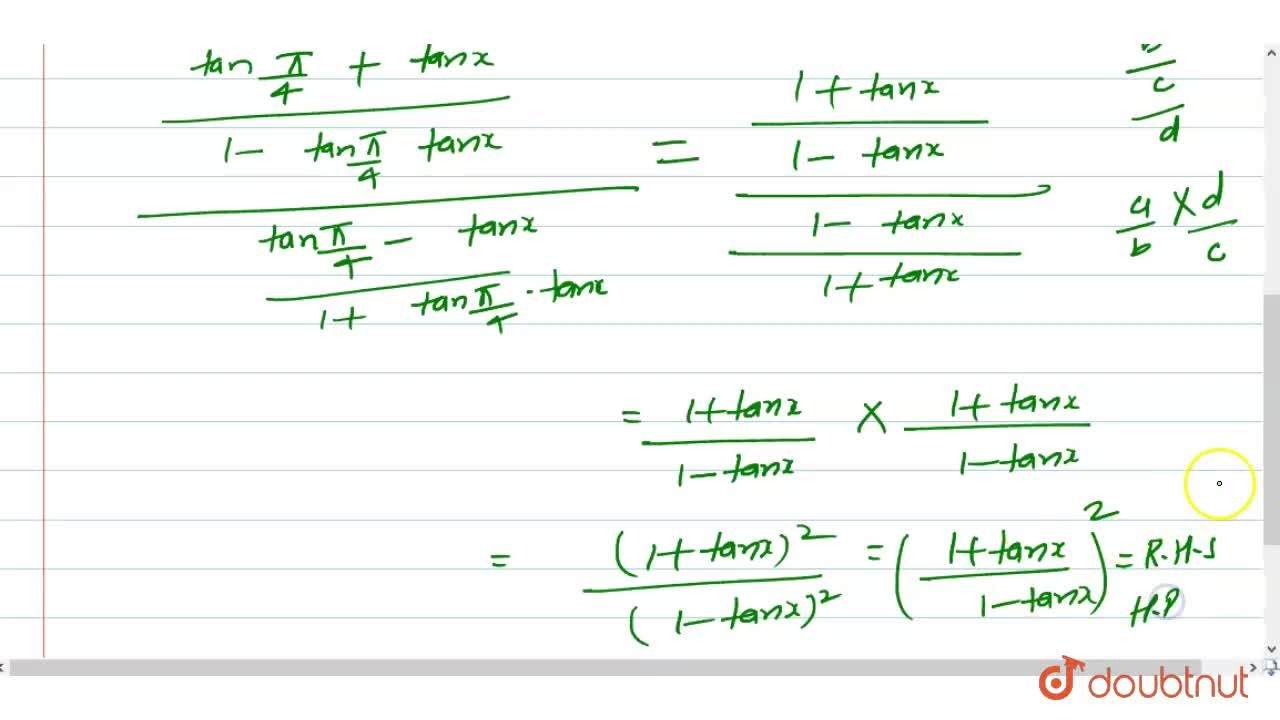
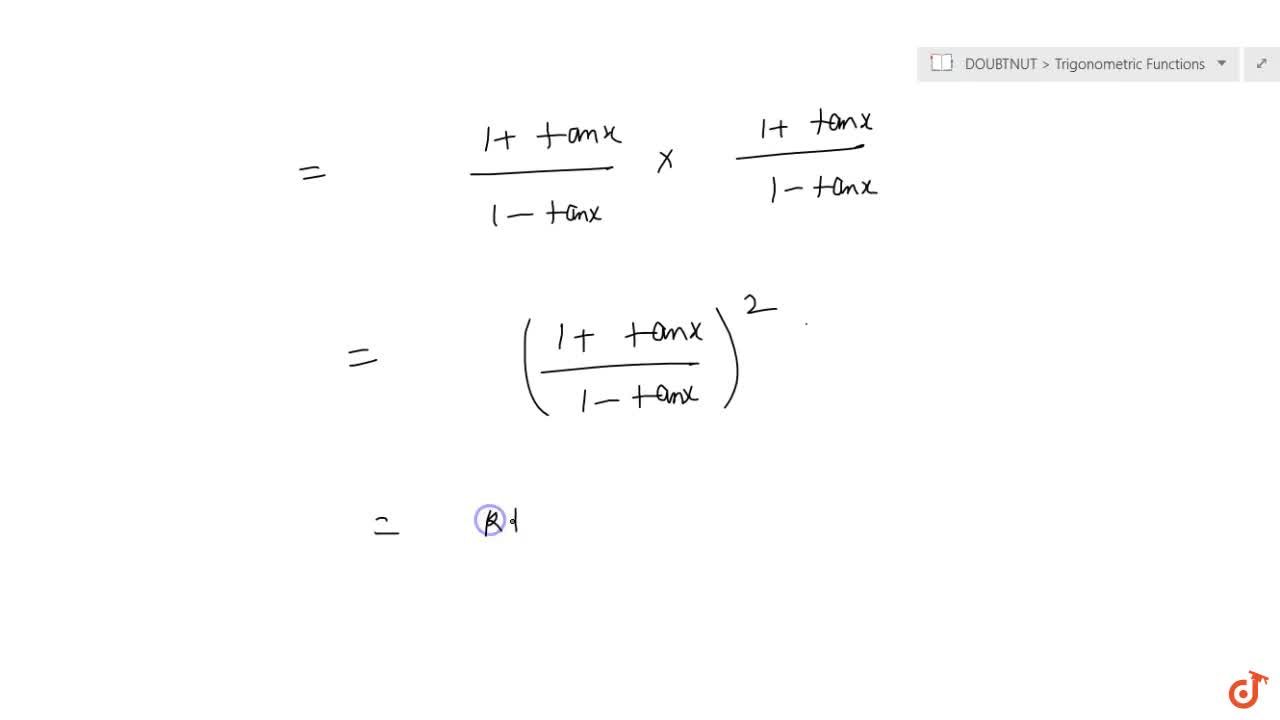
Let F 0 2 R Be Defined As F X Log 2 1 Tan Px 4 Sarthaks Econnect Largest Online Education Community
Example 342 Find the derivative of 625 − x 2 / x in two ways using the quotient rule, and using the product rule Quotient rule d d x 625 − x 2 x = x ( − x / 625 − x 2) − 625 − x 2 ⋅ 1 / ( 2 x) x Note Therefore, we can take the derivative of the given function at the point where f (x) = 3, then take the multiplicative inverse of that result As a simpler example, take the function f ( x)
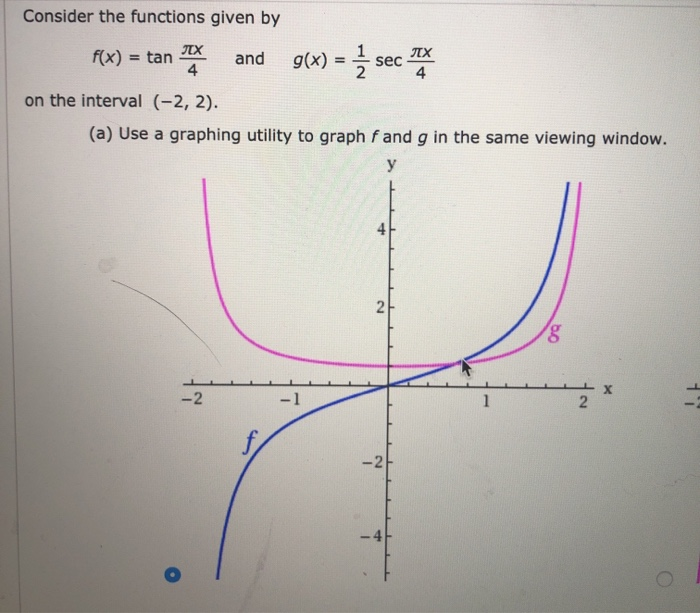
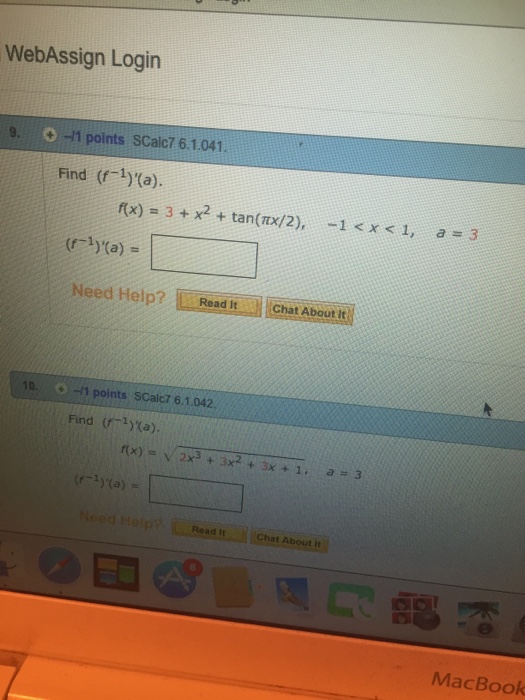
F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5
F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5-Of this last equation are T = 1,2,3 and hence tanπx is Thus for x = 1/2 we have f(17/2) = f(1/2) or 17/2 b = 1/2 so b = 8 on the interval 8 < x < 9 4 Section 102 In Problems 13 through 18X Note that f(−1252) = 1649, which is far from 0 We need to make three more iterations to get reasonably close to the answer 1 Computational Problem The function f(x) = tan(πx)− 6 has a
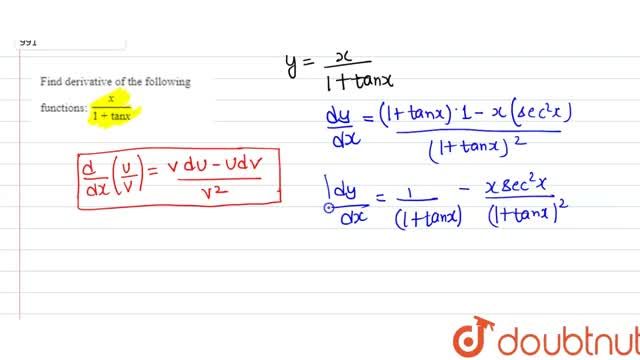
Let F X X Tan Px Where G I F Find The R H D At X K Where K 1 Sarthaks Econnect Largest Online Education Community
Expert's answer Here, F= 2xzixjy^2k F = 2xzi− xj y2k The region V is covered (a) by keeping x and y fixed and integrating from z=x^2 \ to\ z=4 z = x2 to z = 4 (base to top of高数第一章讨论函数f (x)= (1x^2n)/ (1x^2n)x 在x趋向于无穷时的极限答案是当x1时,为x 1年前 1个回答 什么叫函数极限的表现形式同济高数page31有一句话:"由于自变量的变化过程不同,函数Arrow_forward Literature guides Concept explainers Writing
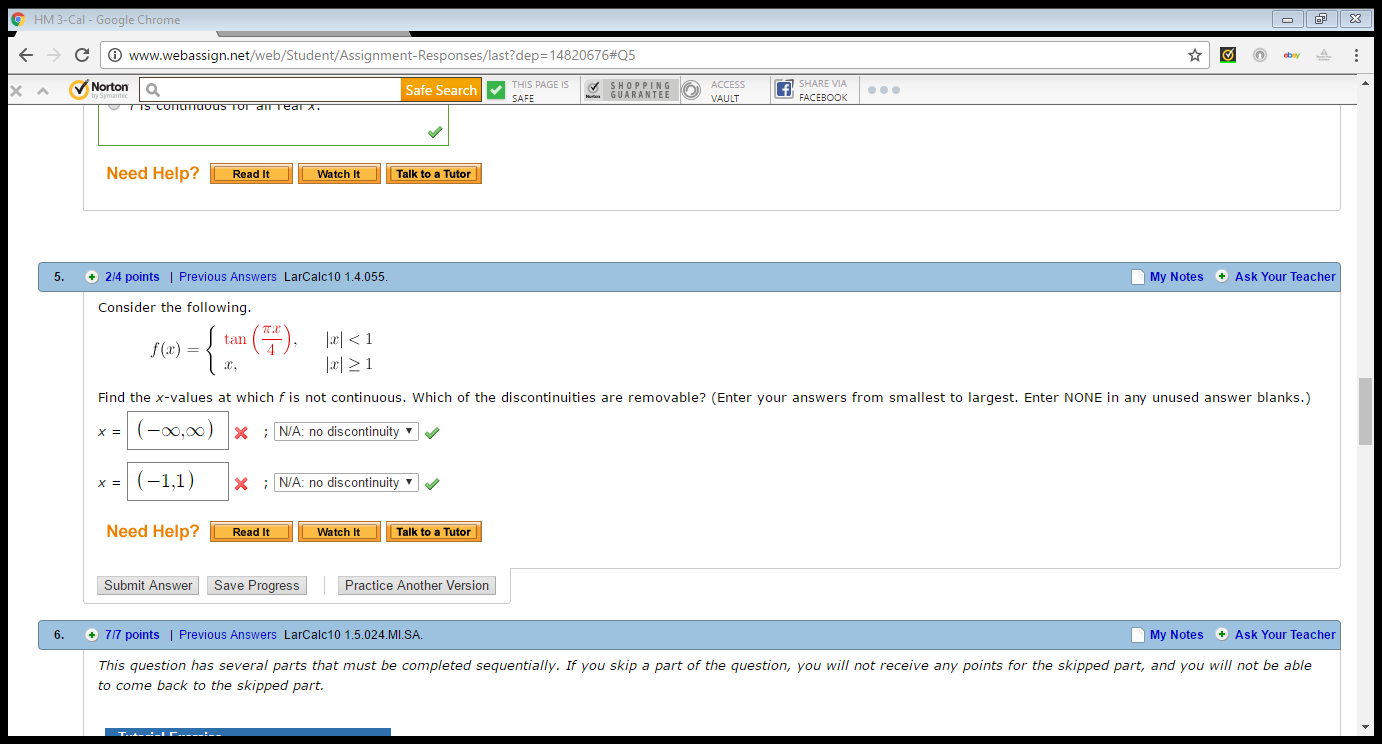
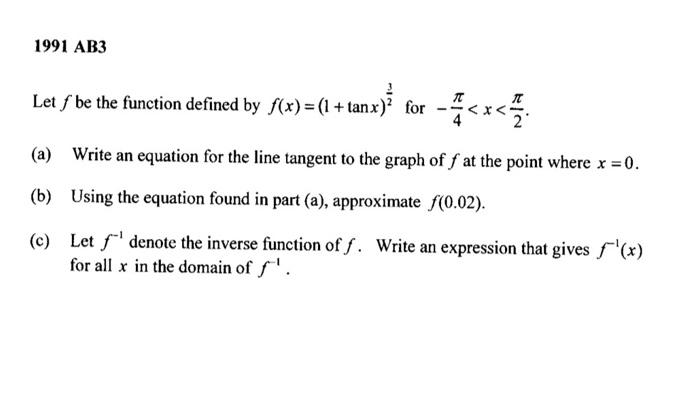
We know that $\ds f'(x)=(1/2)x^{1/2}$, so $\ds f'(g(x))= (1/2)(625x^2)^{1/2}$ Note that this is a two step computation first compute $f'(x)$, then replace $x$ by $g(x)$ Since $g'(x)=2x$ weIf f(x) = sqrt ( 3^x 3^(1x) 2) and g(x) = tan (pi*x) then domain of fog(x) is Maths Relations and Functions NCERT Solutions;This problem has been solved Problem 14E Chapter CH23 Problem 14E The function f (x) = tan πx − 6 has a zero at ( 1 /π) arctan 6 ≈ Let p0
F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 | 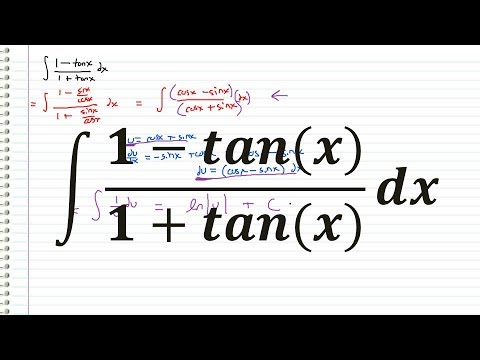 | |
 |  | |
 |  | 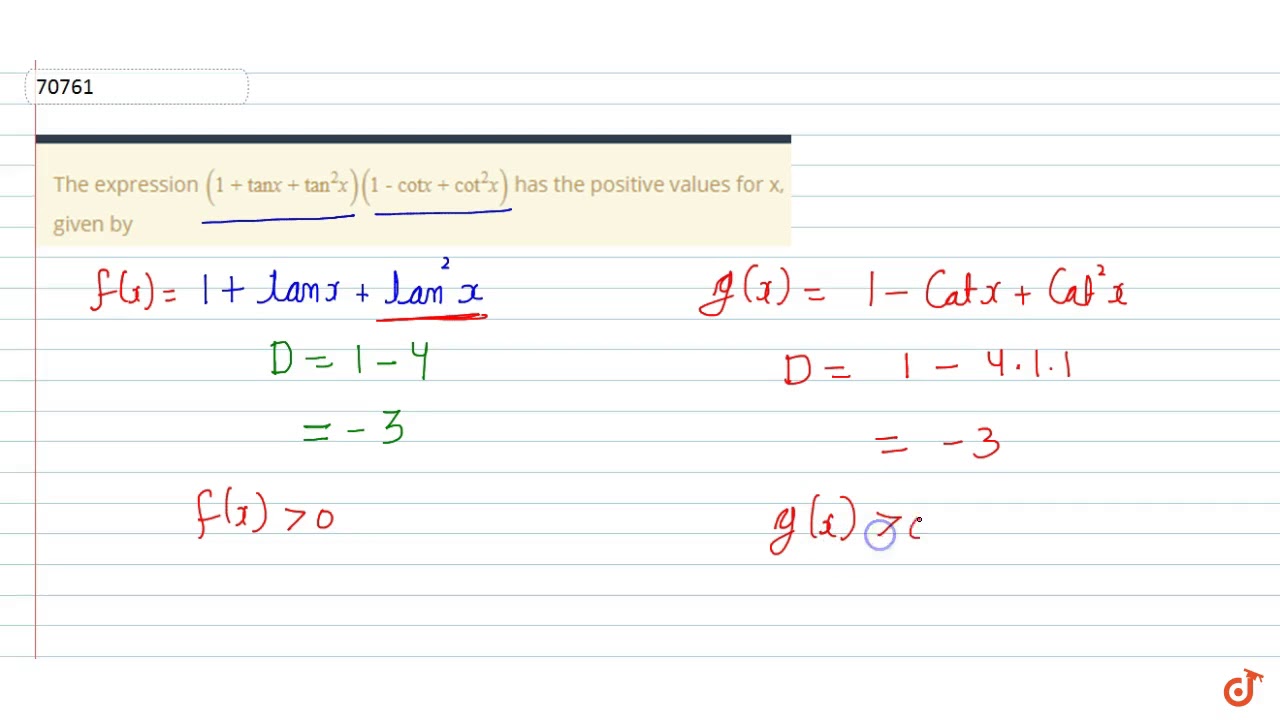 |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 | ||
 |  | |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 | ||
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  |  |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
 |  |  |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
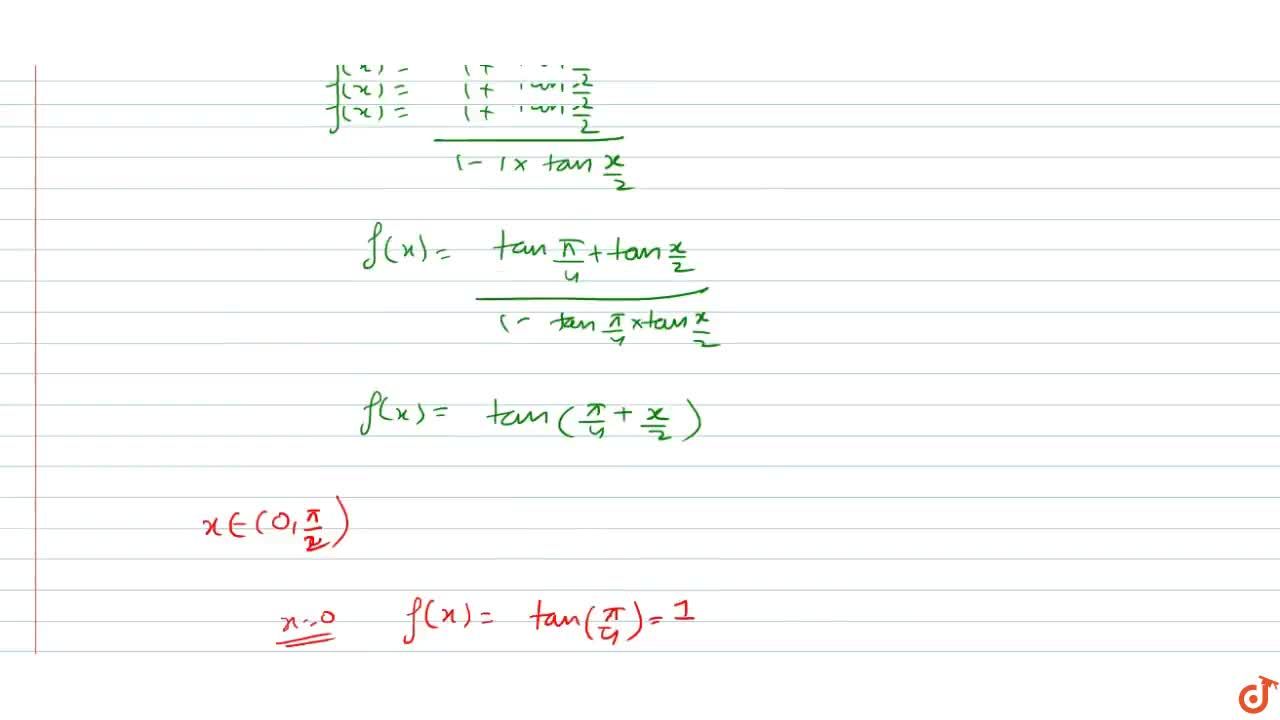 |  | |
 |  | |
 | ||
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  |  |
 |  |  |
「F(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 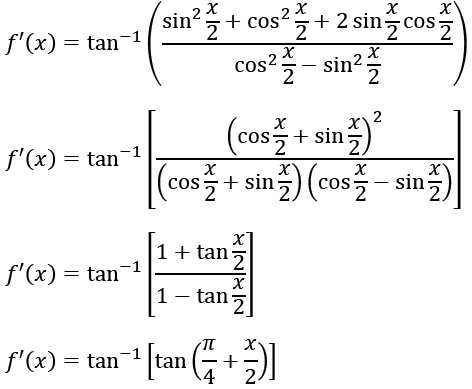 |  |
 |
Calc Test 2 34 1 Write the composite function in the form f (g (x)) Identify the inner function u=g (x) and the outer function y=f (u) Then find the derivative dy/dxObviously, I'll love you forever if you can show me the steps to this one Seriously I appreciate it(and that's tangent to the power of 6) Created by Hillary sciencemathematicsen
Incoming Term: f(x)=(x-1)tanπx/2, f(x) = 9 + x2 + tan πx 2 −1 x 1 a = 9, f(x) = 5 + x2 + tan(πx/2) −1 x 1 a = 5,




No comments:
Post a Comment